Resolution :: SAS/QC(R) 12.1 User's Guide

Resolution

The resolution of a design indicates which effects can be estimated free of other effects. The resolution of a design is generally defined as the smallest order^[32] of the interactions that are confounded with zero. Since having an effect of order confounded with zero is equivalent to having an effect of order n confounded with an effect of order m, the resolution can be interpreted as follows:

If r is odd, then effects of order or less can be estimated free of each other. However, at least some of the effects of order e are confounded with interactions of order e + 1. A design of odd resolution is appropriate when effects of interest are those of order e or less, while those of order e + 1 or higher are all negligible.
If r is even, then effects of order 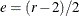or less can be estimated free of each other and are also free of interactions of order e + 1. A design of even resolution is appropriate when effects of order e or less are of interest, effects of order e + 1 are not negligible, and effects of order e + 2 or higher are negligible. If the design uses blocking, interactions of order e + 1 or higher might be confounded with blocks.

In particular, for resolution 5 designs, all main effects and two-factor interactions can be estimated free of each other. For resolution 4 designs, all main effects can be estimated free of each other and free of two-factor interactions, but some two-factor interactions are confounded with each other and/or with blocks. For resolution 3 designs, all main effects can be estimated free of each other, but some of them are confounded with two-factor interactions.

In general, higher resolutions require larger designs. Resolution 3 designs are popular because they handle relatively many factors in a minimal number of runs. However, they offer no protection against interactions. If resources are available, you should use a resolution 5 design so that all main effects and two-factor interactions are independently estimable. If a resolution 5 design is too large, you should use a design of resolution 4, which ensures estimability of main effects free of any two-factor interactions. In this case, if data from the initial design reveal significant effects associated with confounded two-factor interactions, further experiments can be run to distinguish between effects that are confounded with each other in the design. See Example 7.2 for an example.

Many references on fractional factorial designs use Roman numerals to denote resolution of a design—III, IV, V, and so on. A common notation for an orthogonally confounded design of resolution r for k q-level factors in $q^{k-p}$ runs is

$q^{k-p}_ r$

For example, $2^{5-1}_\mr {V}$ denotes a design for five 2-level factors in 16 runs that permits estimation of all main effects and two-factor interactions. This chapter uses Arabic numerals for resolution since these correspond directly to what you specify with the RESOLUTION= option in the MODEL statement.

^[32]The order of an effect is the number of factors involved in it. For example, main effects have order one, two-factor interactions have order two, and so on.