The MULTTEST Procedure

Agresti, A. (2002), Categorical Data Analysis, Second Edition, New York: John Wiley & Sons.

Allison, D. B., Gadbury, G. L., Moonseong, H., Fernández, J. R., Lee, C., Prolla, T. A., and Weindruch, R. (2002), “A Mixture Model Approach for the Analysis of Microarray Gene Expression Data,” Computational Statistics and Data Analysis, 39, 1–20.

Armitage, P. (1955), “Tests for Linear Trend in Proportions and Frequencies,” Biometrics, 11, 375–386.

Benjamini, Y. and Hochberg, Y. (1995), “Controlling the False Discovery Rate: A Practical and Powerful Approach to Multiple Testing,” Journal of the Royal Statistical Society, Series B, 57, 289–300.

Benjamini, Y. and Hochberg, Y. (2000), “On the Adaptive Control of the False Discovery Rate in Multiple Testing with Independent Statistics,” Journal of Educational and Behavioral Statistics, 25, 60–83.

Benjamini, Y., Krieger, A. M., and Yekutieli, D. (2006), “Adaptive Linear Step-up False Discovery Rate Controlling Procedures,” Biometrika, 93, 491–507.

Benjamini, Y. and Yekateuli, D. (2001), “The Control of the False Discovery Rate in Multiple Testing under Dependency,” Annals of Statistics, 29, 1165–1188.

Bickis, M. and Krewski, D. (1986), “Statistical Issues in the Analysis of the Long Term Carcinogenicity Bioassay in Small Rodents: An Empirical Evaluation of Statistical Decision Rules,” Environmental Health Directorate.

Brown, B. W. and Russell, K. (1997), “Methods Correcting for Multiple Testing: Operating Characteristics,” Statistics in Medicine, 16, 2511–2528.

Brown, C. C. and Fears, T. R. (1981), “Exact Significance Levels for Multiple Binomial Testing with Application to Carcinogenicity Screens,” Biometrics, 37, 763–774.

Cochran, W. G. (1954), “Some Methods for Strengthening the Common 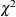Tests,” Biometrics, 10, 417–451.

Dinse, G. E. (1985), “Testing for Trend in Tumor Prevalence Rates: I. Nonlethal Tumors,” Biometrics, 41, 751–770.

Dmitrienko, A., Molenberghs, G., Chuang-Stein, C., and Offen, W. (2005), Analysis of Clinical Trials Using SAS: A Practical Guide, Cary, NC: SAS Institute Inc.

Dudoit, S., Shaffer, J. P., and Boldrick, J. C. (2003), “Multiple Hypothesis Testing in Microarray Experiments,” Statistical Science, 18, 71–103.

Freedman, D. A. (1981), “Bootstrapping Regression Models,” Annals of Statistics, 9, 1218–1228.

Freeman, M. F. and Tukey, J. W. (1950), “Transformations Related to the Angular and the Square Root,” Annals of Mathematical Statistics, 21, 607–611.

Gibson, G. and Wolfinger, R. D. (2004), “Gene Expression Profiling Using Mixed Models,” in A. M. Saxton, ed., Genetic Analysis of Complex Traits Using SAS, 251–278, Cary, NC: SAS Publishing.

Good, I. J. (1987), “A Survey of the Use of the Fast Fourier Transform for Computing Distributions,” Journal of Statistical Computation and Simulation, 28, 87–93.

Heyse, J. and Rom, D. (1988), “Adjusting for Multiplicity of Statistical Tests in the Analysis of Carcinogenicity Studies,” Biometrical Journal, 30, 883–896.

Hochberg, Y. (1988), “A Sharper Bonferroni Procedure for Multiple Significance Testing,” Biometrika, 75, 800–803.

Hochberg, Y. and Benjamini, Y. (1990), “More Powerful Procedures for Multiple Significance Testing,” Statistics in Medicine, 9, 811–818.

Hochberg, Y. and Tamhane, A. C. (1987), Multiple Comparison Procedures, New York: John Wiley & Sons.

Hoel, D. G. and Walburg, H. E. (1972), “Statistical Analysis of Survival Experiments,” Journal of the National Cancer Institute, 49, 361–372.

Holland, B. S. and Copenhaver, M. D. (1987), “An Improved Sequentially Rejective Bonferroni Test Procedure,” Biometrics, 43, 417–424.

Holm, S. (1979), “A Simple Sequentially Rejective Multiple Test Procedure,” Scandinavian Journal of Statistics, 6, 65–70.

Hommel, G. (1988), “A Comparison of Two Modified Bonferroni Procedures,” Biometrika, 75, 383–386.

Hsueh, H., Chen, J. J., and Kodell, R. L. (2003), “Comparison of Methods for Estimating the Number of True Null Hypotheses in Multiplicity Testing,” Journal of Biopharmaceutical Statistics, 13, 675–689.

Lagakos, S. W. and Louis, T. A. (1985), “The Statistical Analysis of Rodent Tumorigenicity Experiments,” in D. B. Clayson, D. Krewski, and I. Munro, eds., Toxicological Risk Assessment, 144–163, Boca Raton, FL: CRC Press.

Liu, W. (1996), “Multiple Tests of a Non-hierarchical Finite Family of Hypotheses,” Journal of the Royal Statistical Society, Series B, 58, 455–461.

Mantel, N. (1980), “Assessing Laboratory Evidence for Neoplastic Activity,” Biometrics, 36, 381–399.

Mantel, N. and Haenszel, W. (1959), “Statistical Aspects of Analysis of Data from Retrospective Studies of Disease,” Journal of the National Cancer Institute, 22, 719–748.

Marcus, R., Peritz, E., and Gabriel, K. R. (1976), “On Closed Testing Procedures with Special Reference to Ordered Analysis of Variance,” Biometrika, 63, 655–660.

Miller, J. J. (1978), “The Inverse of the Freeman-Tukey Double Arcsine Transformation,” The American Statistician, 32, 138.

Osborne, J. A. (2006), “Estimating the False Discovery Rate Using SAS,” in Proceedings of the Thirty-first Annual SAS Users Group International Conference, Cary, NC: SAS Institute Inc.

Pagano, M. and Tritchler, D. (1983), “On Obtaining Permutation Distributions in Polynomial Time,” Journal of the American Statistical Association, 78, 435–440.

Peto, R., Pike, M. C., and Day, N. E. (1980), “Guidelines for Simple, Sensitive Significance Tests for Carcinogenic Effects in Long-Term Animal Experiments,” Long-Term and Short-Term Screening Assays for Carcinogens: A Critical Appraisal.

Press, W. H., Teukolsky, S. A., Vetterling, W. T., and Flannery, B. P. (1992), Numerical Recipes in C: The Art of Scientific Computing, Second Edition, Cambridge: Cambridge University Press.

Sarkar, S. K. and Chang, C.-K. (1997), “The Simes Method for Multiple Hypothesis Testing with Positively Dependent Test Statistics,” Journal of the American Statistical Association, 92, 1601–1608.

Satterthwaite, F. E. (1946), “An Approximate Distribution of Estimates of Variance Components,” Biometrics Bulletin, 2, 110–114.

Schweder, T. and Spjøtvoll, E. (1982), “Plots of P-Values to Evaluate Many Tests Simultaneously,” Biometrika, 69, 493–502.

Shaffer, J. P. (1986), “Modified Sequentially Rejective Multiple Test Procedures,” Journal of the American Statistical Association, 81, 826–831.

Šidák (1967), “Rectangular Confidence Regions for the Means of Multivariate Normal Distributions,” Journal of the American Statistical Association, 62, 626–633.

Simes, R. J. (1986), “An Improved Bonferroni Procedure for Multiple Tests of Significance,” Biometrika, 73, 751–754.

Soper, K. A. and Tonkonoh, N. (1993), “The Discrete Distribution Used for the Log-Rank Test Can Be Inaccurate,” Biometrical Journal, 35, 291–298.

Storey, J. D. (2002), “A Direct Approach to False Discovery Rates,” JRSS-B, 64, 479–498.

Storey, J. D., Taylor, J. E., and Siegmund, D. (2004), “Strong Control, Conservative Point Estimation, and Simultaneous Conservative Consistency of False Discovery Rates: A Unified Approach,” JRSS-B, 66, 187–205.

Storey, J. D. and Tibshirani, R. (2003), “Statistical Significance for Genomewide Studies,” in Proceedings of the National Academy of Sciences of the United States of America, volume 100, 9440–9445.

Turkheimer, F. E., Smith, C. B., and Schmidt, K. (2001), “Estimation of the Number of ’True’ Null Hypotheses in Multivariate Analysis of Neuroimaging Data,” NeuroImage, 13, 920–930.

Westfall, P. H. (2005), “Combining P Values,” in P. Armitage and T. Colton, eds., Encyclopedia of Biostatistics, Second Edition, 987–991, Chichester, UK: John Wiley & Sons.

Westfall, P. H. and Lin, Y. (1988), “Estimating Optimal Continuity Corrections in Run Time,” Proceedings of the Statistical Computing Section.

Westfall, P. H. and Soper, K. A. (1994), “Nonstandard Uses of PROC MULTTEST: Permutational Peto Tests; Permutational and Unconditional t and Binomial Tests,” in Proceedings of the Nineteenth Annual SAS Users Group International Conference, Cary, NC: SAS Institute Inc.

Westfall, P. H., Tobias, R. D., Rom, D., Wolfinger, R. D., and Hochberg, Y. (1999), Multiple Comparisons and Multiple Tests Using the SAS System, Cary, NC: SAS Institute Inc.

Westfall, P. H. and Wolfinger, R. D. (1997), “Multiple Tests with Discrete Distributions,” The American Statistician, 51, 3–8.

Westfall, P. H. and Wolfinger, R. D. (2000), “Closed Multiple Testing Procedures and PROC MULTTEST,” Observations.

Westfall, P. H. and Young, S. S. (1989), “P-Value Adjustments for Multiple Tests in Multivariate Binomial Models,” Journal of the American Statistical Association, 84, 780–786.

Westfall, P. J. and Young, S. S. (1993), Resampling-Based Multiple Testing, New York: John Wiley & Sons.

Yates, F. (1984), “Tests of Significance for 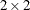Contingency Tables,” Journal of the Royal Statistical Society.

Yekateuli, D. and Benjamini, Y. (1999), “Resampling-Based False Discovery Rate Controlling Multiple Test Procedures for Correlated Test Statistics,” Journal of Statistical Planning and Inference, 82, 171–196.