The NESTED Procedure
A random-effects model for data from a completely nested design with n factors has the general form
where
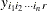
-
is the value of the dependent variable observed at the rth replication with factor j at level
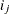 , for
, for 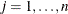 .
.
-
is the overall (fixed) mean of the sampled population.
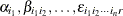
-
are mutually uncorrelated random effects with zero means and respective variances
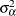 ,
, 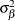 , …,
, …,  .
.
