The SURVEYLOGISTIC Procedure

Adjustments to the Variance Estimation

The factor $(n-1)/(n-p)$ in the computation of the matrix $\widehat{\mb {G}}$ reduces the small sample bias associated with using the estimated function to calculate deviations (Morel, 1989; Hidiroglou, Fuller, and Hickman, 1980). For simple random sampling, this factor contributes to the degrees-of-freedom correction applied to the residual mean square for ordinary least squares in which p parameters are estimated. By default, the procedure uses this adjustment in Taylor series variance estimation. It is equivalent to specifying the VADJUST=DF option in the MODEL statement. If you do not want to use this multiplier in the variance estimation, you can specify the VADJUST=NONE option in the MODEL statement to suppress this factor.

In addition, you can specify the VADJUST=MOREL option to request an adjustment to the variance estimator for the model parameters $\hat{\btheta }$ , introduced by Morel (1989):

$\widehat V(\hat{\btheta })= \widehat{\mb {Q}}^{-1} \widehat{\mb {G}} \widehat{\mb {Q}}^{-1} + \kappa \lambda \widehat{\mb {Q}}^{-1}$

where for given nonnegative constants $\delta$ and $\phi$ ,

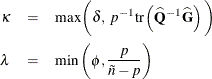
$\begin{eqnarray*} \kappa & = & \textrm{max} \biggl (\delta , \, \, p^{-1}\mbox{tr}\left( \widehat{\mb {Q}}^{-1}\widehat{\mb {G}}\right) \biggl ) \\ \lambda & = & \textrm{min} \left(\phi , \displaystyle {\frac{p}{\tilde n-p}} \right) \end{eqnarray*}$

The adjustment $\kappa \lambda \widehat{\mb {Q}}^{-1}$ does the following:

reduces the small sample bias reflected in inflated Type I error rates
guarantees a positive-definite estimated covariance matrix provided that 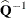$\widehat{\mb {Q}}^{-1}$ exists
is close to zero when the sample size becomes large

In this adjustment, $\kappa$ is an estimate of the design effect, which has been bounded below by the positive constant $\delta$ . You can use DEFFBOUND= $\delta$ in the VADJUST=MOREL option in the MODEL statement to specify this lower bound; by default, the procedure uses $\delta =1$ . The factor $\lambda$ converges to zero when the sample size becomes large, and $\lambda$ has an upper bound $\phi$ . You can use ADJBOUND= $\phi$ in the VADJUST=MOREL option in the MODEL statement to specify this upper bound; by default, the procedure uses $\phi =0.5$ .